Project 3

Vibrational modes of glasses: The effects of disorder and nonlinearity

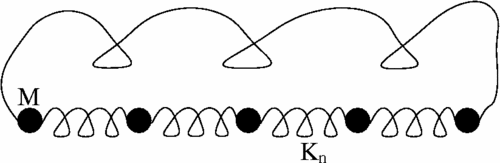
A linear chain of point masses coupled by harmonic springs is a standard model used to introduce various concepts of solid state physics.

An illustration of a linear chain of masses with periodic boundary conditions which effectively connect first and last mass by a spring.

The ordered chain has sinusoidal standing wave normal modes (if the ends are fixed) or traveling wave normal modes (if the ends are connected in a ring via periodic or, the so-called, Born-Von Karman boundary conditions). Ballistically (i.e., without any scattering) propagating wave packets can be built from these normal modes, and illustrate the mechanism of heat propagation in insulating crystals. When disorder is introduced in the chain, as simulated by random spring constants $K_{n}$ in the Figure above (or random masses), qualitatively new effects arise: on length scales longer than the localization length, energy is trapped in few atoms unless there are nonlinear forces [for more details on localization in disordered chains see References below].

Part I for both PHYS460 and PHYS660 students

Consider a chain of $L=12000$ atoms with periodic boundary conditions. Its harmonic springs are disordered so that $K_{n}=K_{0}(1+r_{n})$, where $r_{n}$ is chosen by a random number generator and is uniformly distributed in the interval $(-b,b)$, with $b=0.3$. To simulate hypothetical linear silicon chain (the silicon atom mass is M), choose $K_{0}=10.6\ \mathrm {eV/\mathrm {\AA} ^{2}}$ which specifies the maximum vibrational frequency $\hbar \omega _{\mathrm {max} }=2{\sqrt {K_{0}/M}}\approx 80\ \mathrm {meV}$ when there is no disorder (i.e., when $b=0$).

Using exact numerical diagonalization of the dynamical matrix, find vibrational frequencies and eigenstates (a system of 12000 atoms should be sufficiently large to ensure that the conclusions are not affected by the finite-size effects).

(a) From the eigenfrequencies compute the vibrational density of states (DOS) defined as

$N(\omega )={\frac {1}{L}}\sum _{i}\delta (\omega -\omega _{i})$

where the $\delta$-function is broadened into a box function (i.e., you just have to bin the eigenfrequencies into equally spaced bins in the obtained range of eigenfrequencies, and then divide the final number in each bin by $L$ and the size of the bin itself). Plot DOS for both clean and disordered chain as a function of frequency.

(b) To understand the difference between propagating and localized modes, compute the participation ratio (which tells us how many atoms participate in the vibration of a particular mode) for an eigenstate $e_{i}(a)$:

$P_{i}=\left(\sum _{a=1}^{L}[e_{i}(a)]^{4}\right)^{-1}$

From the plot of $P_{i}$ vs. $\omega _{i}$ locate the mobility edge as the frequency where a transition from extended to localized states occurs (roughly where $P_{i}\leq 10$).

Part II for PHYS660 students only

In addition to (a), perform disorder averaging to obtain average values of quantities introduced in (a) over an ensemble of chains. Your ensemble should contain at least 10 chains of 12000 atoms with different realizations of its disordered harmonic springs. After you have obtained eigenfrequencies and eigenstates for all of these chains, you have to: create bins on the frequency axis; sum the DOS or participation ratio of different chains falling in the same bin; and, finally, divide those numbers by the number of chains to get arithmetic average values for the DOS and the participation ratio.

REFERENCES

Vibrational eigenmodes

• P. B. Allen and J. Kelner, Evolution of a vibrational wave packet on a disordered chain, Am. J. Phys. 66, 497 (1998). [PDF]

• J. Fabian, Decay of localized vibrational states in glasses: A one-dimensional example, Phys. Rev. B 55, R3328 (1997). [PDF]

50th Anniversary of the Fermi-Pasta-Ulam Problem

• Focus issue of "Chaos": THE "FERMI-PASTA-ULAM" PROBLEM-THE FIRST 50 YEARS.
• S. Flach, M. V. Ivanchenko, O. I. Kanakov, and K. G. Mishagin, Periodic orbits, localization in normal mode space, and the Fermi-Pasta-Ulam problem, Am. J. Phys. 76, 453 (2008). [PDF].
• Fermi-Pasta-Ulam nonlinear lattice oscillations, T. Dauxois and S. Ruffo (2008), Scholarpedia, 3(8):5538.